Electric Metal Race Tracks

Galton (second, first) Slideshow

This year I am nominated in high school, and my plan to make crafts for the college program is modified. Conduct visual and tactile tools for teaching math in high school seems a little harder, and limit off-topic, the program going towards more abstraction. However, for some lessons, like geometry in space, this approach can be interesting. I made this object a few years ago, but I shall certainly need this year, I recovered (Thanks Jacques). A Galton board, named after its inventor, Sir Francis Galton . Two removable rods up and down to release the ball. I used hex head screws, to have a probability 1 / 2 for each side. The results are correct, I wanted to use barriers to make a circular cross section for another board (the wooden dowels), but the results are very disappointing (the balls are very often on the sides). With the screws, once the board placed on a balanced place, we have results rather correct. is the first object I tinkered, the biggest problem he has is that the equipment cost me quite expensive, especially screws 21 (around 25 €, I remember correctly)



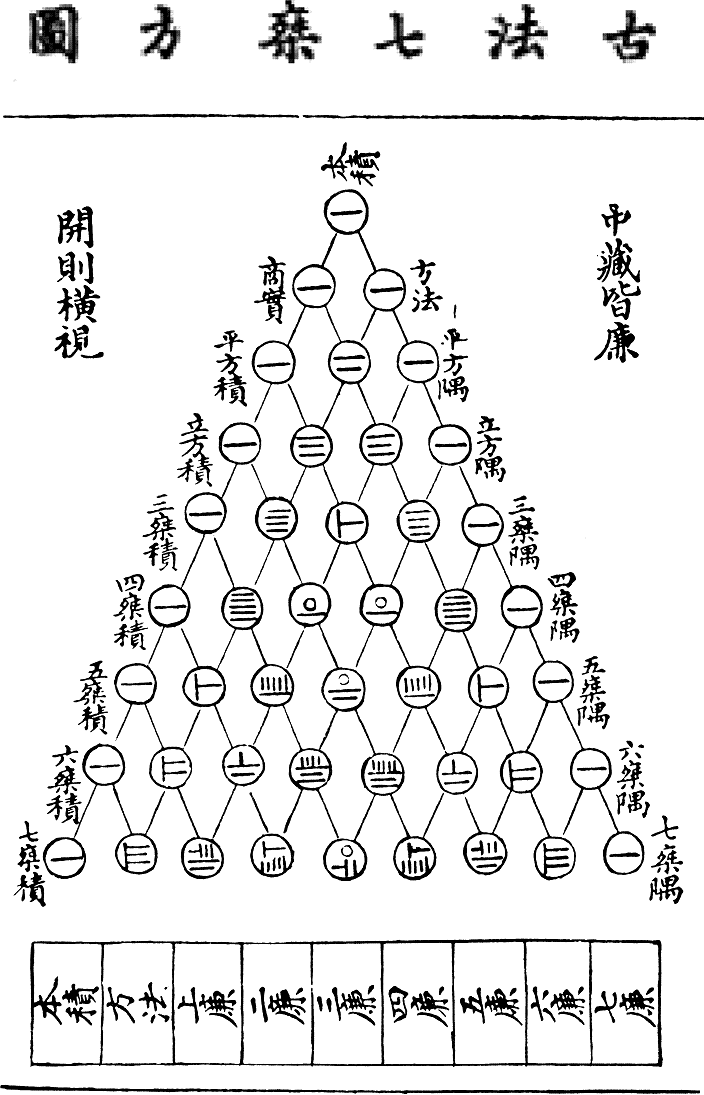
With the new programs of a second, an interesting place is given to algorithms, and I plunged with delight into the lineup of small programs. I used to write software scratch, because the new programs we offer second to discover this program. I was amused to perform a simulation of the Galton board. One of the nice features of Scratch is the opportunity to share our programs to other users, who can download and use and modify if they wish. Here is my simulation (take a value less than 150, please) Activity related to the Galton board: After a run of sixty balls, establish a frequency table for each column. How to explain this bell curve? Throw ball: where the ball will she fall? Why does the ball lands it more often in the middle column? Property: the probability for the ball falling into a column is proportional to the number of paths that lead to this column. We must therefore count for each column the number of paths leading to it. A path notation: For each obstacle, the ball will either left or right. Noting that the G and D to move left or right for each obstacle, draw the path GGDDGGGD. Where the ball lands after she this way? Give another path that leads to the same column. How many are there of road that leads into the right-most column? What is its code? By studying the number of opportunities in each barrier 1) on the edges 2) on an obstacle located on the banks, we come to realize that we can get closer and closer the number of paths leading to each step. We get Pascal's triangle, known as the 14th century Chinese